Point particle

Standard model of particle physics
Feynmann Diagram Gluon Radiation.svg
Standard Model

A point particle (ideal particle[1] or point-like particle, often spelled pointlike particle) is an idealized object heavily used in physics. Its defining feature is that it lacks spatial extension: being zero-dimensional, it does not take up space.[2] A point particle is an appropriate representation of any object whose size, shape, and structure is irrelevant in a given context. For example, from far enough away, an object of any shape will look and behave as a point-like object. Particular "types" of point particles include point masses and point charges, point particles whose only attributes are their mass and charges respectively.[3]

Sometimes due to specific combinations of properties extended objects behave as point-like even in their immediate vicinity. For example, spherical objects interacting in 3-dimensional space whose interactions are described by the inverse square law behave in such a way as if all their matter were concentrated in their geometric centers. In Newtonian gravitation and classical electromagnetism, for example, the respective fields outside of a spherical object are identical to those of a point particle of equal charge/mass located at the center of the sphere.[4][5]

In particle physics, "point particle" is synonymous with "elementary particle", which is defined as a particle without structure or, equivalently, as a particle which is not made up from component parts. According to the Standard Model of fundamental particles and forces, quarks, leptons and the (non-composite) vector bosons are point particles in this sense. There is no experimental evidence for any of the elementary particles having spatial extent, and so they are usually considered to be point particles in the more general sense too (at least to the limited extent that the concept of a "particle" is meaningful in quantum field theory.)[6]

Contents

History of the point particle

Around 600 B.C. Thales, of Miletus, in ancient Greece, hypothesized that there was a single fundamental building block of all matter. However, he incorrectly thought this to be water. Around 450 B.C. Democritus hypothesized there was a single un-cuttable particle underlying all matter, which he called atomos. In modern times, as early as 1878, researchers moved closer to the discovery of a fundamental (point) particle when George Johnstone Stoney observed a unit of charge missing from an ionized atom. Stoney named this unit of charge the "electron".[7]

In 1897, the electron was the first point particle discovered. In the 1890s there was intense activity by physicists in England, Scotland, Germany and the United States, studying the electrical discharges of gases. The one who successfully discovered the electron as a constituent of the atom was J.J. Thomson. "The discovery of the electron was announced during the course of Thomson's evening lecture to the Royal Institution on Friday, April 30, 1897." In 1898, using a cloud chamber he measured the electric charge of his cathode rays. He was able to measure both the charge and mass of the electron.[8][9]

Today, it is known that the electron is one of the most stable elementary (point) particles. It will exist for a long time (believed created at Big Bang), whereas most other elementary particles last only tiny fractions of a second. The estimated lifetime of an electron is at least 4.6×1026 years, more than a quadrillion times the current age of the Universe.[10][11]

The quark is also a point particle (elementary particle) of which all matter is composed. In 1964, Murray Gell-Mann of Caltech, and George Zweig a post doc of the same institution, proposed the existence of quarks. Gell Mann named the particle "quark" while Zweig called them "aces".[12] In 1968 the Stanford Linear Accelerator Center (SLAC) did deep inelastic electron-nucleon scattering experiments which proved the existence of quarks. The SLAC conducted these types of scattering experiments between 1967 and 1973.[13][14]

The quarks combine in combinations of three to make up the proton and neutron in atomic nuclei. The electrons and the quarks, when combined in the configuration known today as atoms, are the fundamental components of all the elements, hence all matter, everywhere in the Universe. In addition, quarks combine with other quarks or anti quarks, to form all the other hadrons.[14]

Point particle properties

In a closed physical system the particle properties are conserved.

Electron

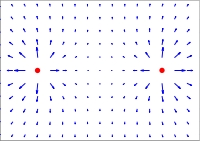
Illustration of the electric vector field surrounding two point charges.
Main article: Electron

Although the electron is considered to be without dimension, it does have mass (rest mass), energy, spin (angular momentum), and electric charge:[15] The electron, as a particle with spin, mass, and charge, but no dimension, is counterintuitive to the human perspective and experience.[16]

The electron is part of the lepton family, and it has no internal structure. This is in contrast to the muon, another lepton, which will spontaneously decay into less massive particles. The muon decays into an electron, a neutrino and an antineutrino, with a mean lifetime of 2.2 micro-seconds (2.2×10−6 s).

Moreover, no internal structure is in contrast to the neutron and the proton, which are made up of quarks, and in contrast to the atom, for example, which is composed of nucleons and electrons.[6][11]

Quark

Main article: Quark
A proton, composed of two up quarks and one down quark. The strong force has three types of charges, the so called red, green and the blue. Note that the choice of red, green or blue for the up or down quark is arbitrary. In addition, the "color charge" is thought of as circulating between the three quarks.

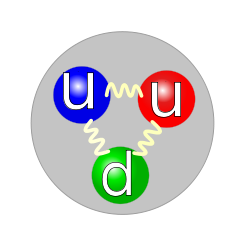
The quark is the principal building block of all hadrons, and, also, has no internal structure. Quarks occur only in groups of three or two. As constituents of neutrons and protons, they occur in groups of three. They have an electric charge, like the electron, but they also have a charge known as the "strong force". The strong force binds the protons and neutrons of the atoms together.

Quarks come in six different types known as flavors: up, down, charm, strange, top, and bottom. Up quarks and Down quarks have the least mass. These are also the most common, and the most stable quarks in the universe, especially since they make up the proton and neutron, or essentially, the nucleus of the atom. The heavier quarks are involved in a process called a weak interaction. The weak interaction is called a particle decay. In the decay process the four heavier quarks; Charm, Strange, Top, and Bottom quark, rapidly transform from a higher mass state to a lower mass state. This lower mass state is the up quark and down quark. Hence, because of this, the Up quark and Down quark are actually the most stable, and these quarks make up 99.5% of the mass of every atom; electrons make up the remaining mass. Existing as constituents of the proton, the up and down quark have an expected lifetime of 2.1×1029 years.[17][18] The different variety of quarks also occur, in groups of three or two, in other, shorter lived composite particles.

See the following main articles: Hadron, Baryon, Meson, and Quark.

In 2003 a controversy developed over the existence of a composite particle consisting of five quarks, called the pentaquark. In 2006, high energy experiments were targeted at proving or disproving the pentaquark. The review of previous pentaquark experiments caused researchers to question their results. After amassing more data, that produced more accurate statistics, the proof for the pentaquark was found to be null.[19]

Vector bosons

Vector bosons are also considered point particles. In particle physics, a vector boson is a boson with the spin quantum number equal to 1. The vector bosons that are considered to be elementary particles are the gauge bosons or, the force carriers of fundamental interactions.

These are part of the Standard Model, and there are three kinds of gauge bosons:

  1. photons,
  2. W and Z bosons, and
  3. gluons.[20]

Each corresponds to one of the three Standard Model interactions: photons are gauge bosons of the electromagnetic interaction, W and Z bosons carry the weak interaction, and the gluons carry the strong interaction. Due to color confinement, isolated gluons do not occur at low energies. What could result instead are massive glueballs (as of 2008, these are not widely confirmed experimentally). In a quantized gauge theory, gauge bosons are quanta of the gauge fields.

Photon

Photon streams on a light bench.
Main article: Photon

The photon is also described as a point particle. In physics, a photon is an elementary particle, the quantum of the electromagnetic field and the basic "unit" of light and all other forms of electromagnetic radiation. Like all elementary particles, photons are governed by quantum mechanics and will exhibit wave-particle duality – they exhibit properties of both waves and particles. The modern concept of the photon was developed gradually by Albert Einstein to explain experimental observations that did not fit the classical wave model of light. In particular, the photon model accounted for the frequency dependence of light's energy, and explained the ability of matter and radiation to be in thermal equilibrium. It also accounted for other anomalous observations constituent to the classical model of physics.

The photon is massless,[21] has no electric charge,[22] and does not decay spontaneously in empty space. Photons are emitted in many natural processes. For example, when a charge is accelerated it emits synchrotron radiation. During a molecular, atomic or nuclear transition to a lower energy level, photons of various energy will be emitted, from infrared light to gamma rays. A photon can also be emitted when a particle and its corresponding antiparticle are annihilated (see Electron-positron annihilation for an example). In empty space, the photon moves at c (the speed of light).[23]

W and Z bosons

Main article: W and Z bosons

The W and Z bosons are carrier particles that mediate the weak nuclear force, much like the photon is the carrier particle for the electromagnetic force. The W bosons are best known for its role in nuclear decay. Consider, for example, the beta decay of cobalt-60, an important process in supernova explosions. The exchange of a Z boson between particles, called a neutral current interaction, therefore leaves the interacting particles unaffected, except for a transfer of momentum. Unlike beta decay, the observation of neutral current interactions requires huge investments in particle accelerators and detectors, such as are available in only a few high-energy physics laboratories in the world.

Point mass

An example of a point mass graphed on a grid. The grey mass can be simplified to a point mass (the black circle). It becomes practical to represent point mass as small circle, or dot, as an actual point is invisible.

Point mass (pointlike mass) is an idealistic term used to describe either matter which is infinitely small, or an object which can be thought of as infinitely small. This concept in terms of size is similar to that of point particles, however unlike point particles the object need only be considered infinitely small.

Application

Physics

A common use for point mass lies in the analysis of the gravitational force fields. When analyzing the gravitational forces in a system, it becomes impossible to account for every unit of mass individually. When none of the objects in the system have overlapping center of mass circumferences, it is possible to consider of the object as a zero-dimensional point mass.

Mathematics

A point mass in statistics is a discontinuous segment in a probability distribution. To calculate such point mass, an integration is carried out over the entire range of the random variable, on the probability distribution of the continuous part. After equating this integral to 1, the point mass can be found by further calculation.

Point charge

A point charge is an idealized model of a particle which has an electric charge. A point charge is an electric charge at a mathematical point with no dimensions.

The fundamental equation of electrostatics is Coulomb's law, which describes the electric force between two point charges. The electric field associated with a classical point charge increases to infinity as the distance from the point charge decreases towards zero making energy (thus mass) of point charge infinite. In quantum electrodynamics, developed in part by Richard Feynman, the mathematical method of renormalization eliminates the infinite divergence of the point charge.

Earnshaw's theorem states that a collection of point charges cannot be maintained in an equilibrium configuration solely by the electrostatic interaction of the charges.

See also

Notes and references

Notes

  1. ↑ H.C. Ohanian, J.T. Markert (2007), p. 3
  2. ↑ F.E. Udwadia, R.E. Kalaba (2007), p. 1
  3. ↑ R. Snieder (2001), pp. 196–198
  4. ↑ I. Newton, I.B Cohen, A. Whitmann (1999), p. 956 (Proposition 75, Theorem 35)
  5. ↑ I. Newton, A. Motte, J. Machin (1729), p. 270–271
  6. ↑ 6.0 6.1 C. Quigg (2009)
  7. ↑ L. Lederman, D. Teresi (1993), pp. x, 35, 41, 135
  8. ↑ L. Lederman, D. Teresi (1993), pp. xii, 139
  9. ↑ "J.J. Thomson – Biography". The Nobel Foundation. http://nobelprize.org/nobel_prizes/physics/laureates/1906/thomson-bio.html. Retrieved 2009-06-28. 
  10. ↑ W.-M. Yao et al. (2006)
  11. ↑ 11.0 11.1 C. Amsler et al. (2009a)
  12. ↑ M.Y. Han (1999), p. 102
  13. ↑ M. Riordan (1992)
  14. ↑ 14.0 14.1 Encyclopædia Britannica (2009)
  15. ↑ One eV/c2, the mass equivalent of one electronvolt of energy.
  16. ↑ L. Lederman, D. Teresi (1993), pp. 141–144
  17. ↑ S.L. Glashow (2009)
  18. ↑ C. Amsler et al. (2009b)
  19. ↑ K. Carter (2006)
  20. ↑ M. Veltman (2003)
  21. ↑ The mass of the photon is believed to be exactly zero, based on experiment and theoretical considerations described in the article. Some sources also refer to the "relativistic mass" concept, which is just the energy scaled to units of mass. For a photon with wavelength λ or energy E, this is h/λc or E/c2. This usage for the term "mass" is no longer common in scientific literature. Further info: M. Austern, S. Chase, P. Gibbs, D. Koks (2008)
  22. ↑ V.V. Kobychev, S.B. Popov (2005)
  23. ↑ M. Alonso, E.J. Finn (1968), Section 1.6

Bibliography

Further reading

External links